Mathematics
Describe the locus of a point at a distant 3 cm from a fixed point.
Locus
7 Likes
Answer
Steps of construction :
Let fixed point be A and B be any point on circle.
- Taking fixed point (A) as center draw a circle of radius (AB) = 3 cm.
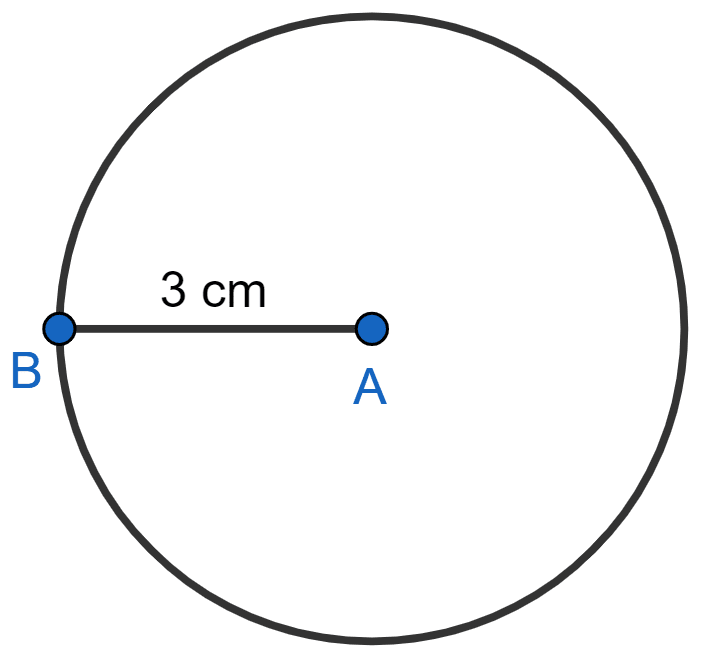
The locus of a point which is 3 cm away from a fixed point is circumference of a circle whose radius is 3 cm and the fixed point is the center of the circle.
Answered By
6 Likes
Related Questions
AB is a line segment and P is a moving point that moves in such a way that it is always equidistant from AB. The locus of point P is the line which :
is parallel to AB and through point P.
is perpendicular to AB through point P.
is perpendicular bisector of AB.
passes through the mid-point of AB.
A point P moves in such a way that it is at a distance less than or equal to 5 cm from a fixed point O. The locus of point P is :
a circle with radius 5 cm.
a circle with OP as radius.
a circle with diameter of 10 cm.
a circle of radius 5 cm and the fixed point O as its center.
Describe the locus of points at a distance 2 cm from a fixed line.
Describe the locus of the center of a wheel of a bicycle going straight along a level road.