Mathematics
Construct a rhombus PQRS whose diagonals PR, QS are 8 cm and 6 cm respectively. Find by construction a point X equidistant from PQ, PS and equidistant from R, S. Measure XR.
Answer
Steps of construction :
Draw QS = 6 cm and PR = 8 cm as diagonals. Join the points to form rhombus PQRS.
Since, diagonals of rhombus bisects vertices, hence, PR is angular bisector of SPQ.
Draw CD, the perpendicular bisector of RS.
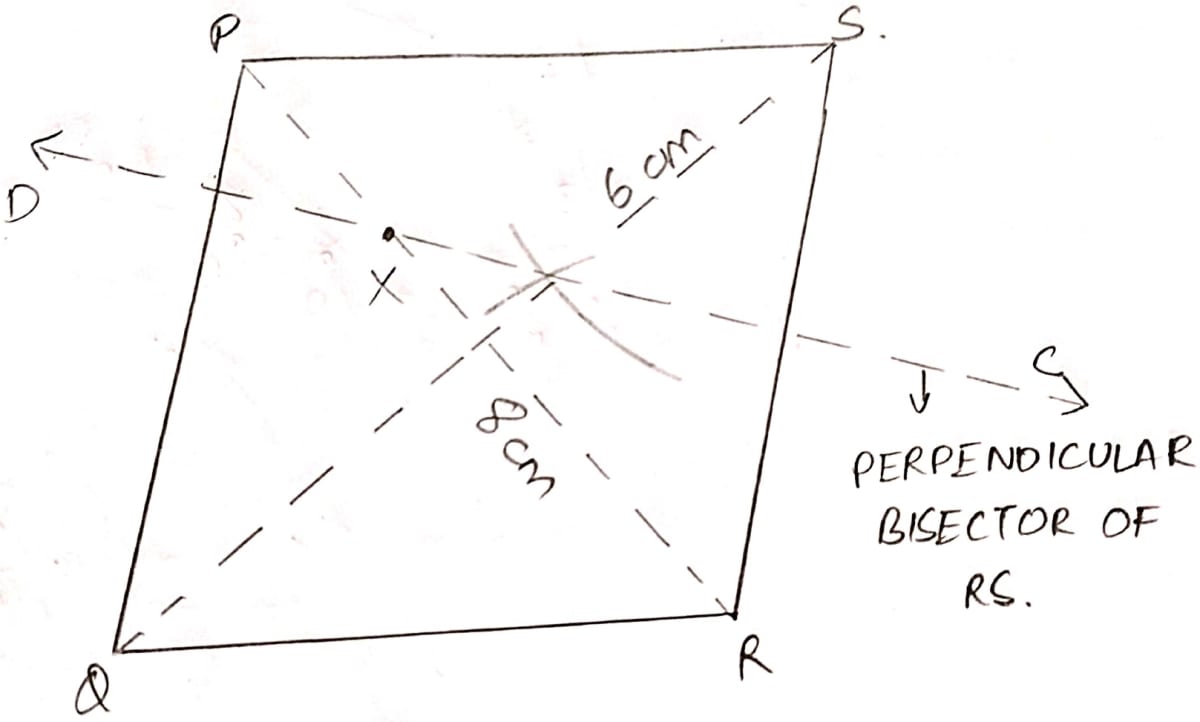
The intersection of CD and PR is the point X which satisfies both i.e. it is equidistant from PQ, PS and R and S also.
On measuring we get, XR = 3.15 cm.
Related Questions
AB and CD are two intersecting lines. Find the position of a point which is at a distance of 2 cm from AB and 1.6 cm from CD.
Two straight roads PQ and PK cross each other at P at an angle of 75°. S is a stone on the road PQ, 800 m from P towards Q. By drawing a figure to scale 1 cm = 100m, locate the position of a flag staff X, which is equidistant from P and S, and is also equidistant from the roads.
Without using set square or protractor, construct the parallelogram ABCD in which AB = 5.1 cm, the diagonal AC = 5.6 cm and the diagonal BD = 7 cm. Locate the point P on DC, which is equidistant from AB and BC.
By using ruler and compasses only, construct a quadrilateral ABCD in which AB = 6.5 cm, AD = 4 cm and ∠DAB = 75°. C is equidistant from the sides AB and AD, also C is equidistant from the points A and B.