Mathematics
As observed from the top of a 80 m tall light house, the angles of depression of two ships on the same side of the light house in horizontal line with its base are 30° and 40° respectively. Find the distance between the two ships. Give your answer correct to nearest meter.
Heights & Distances
38 Likes
Answer
Let AB be the tower of length 80 m and the ships be at point C and D.
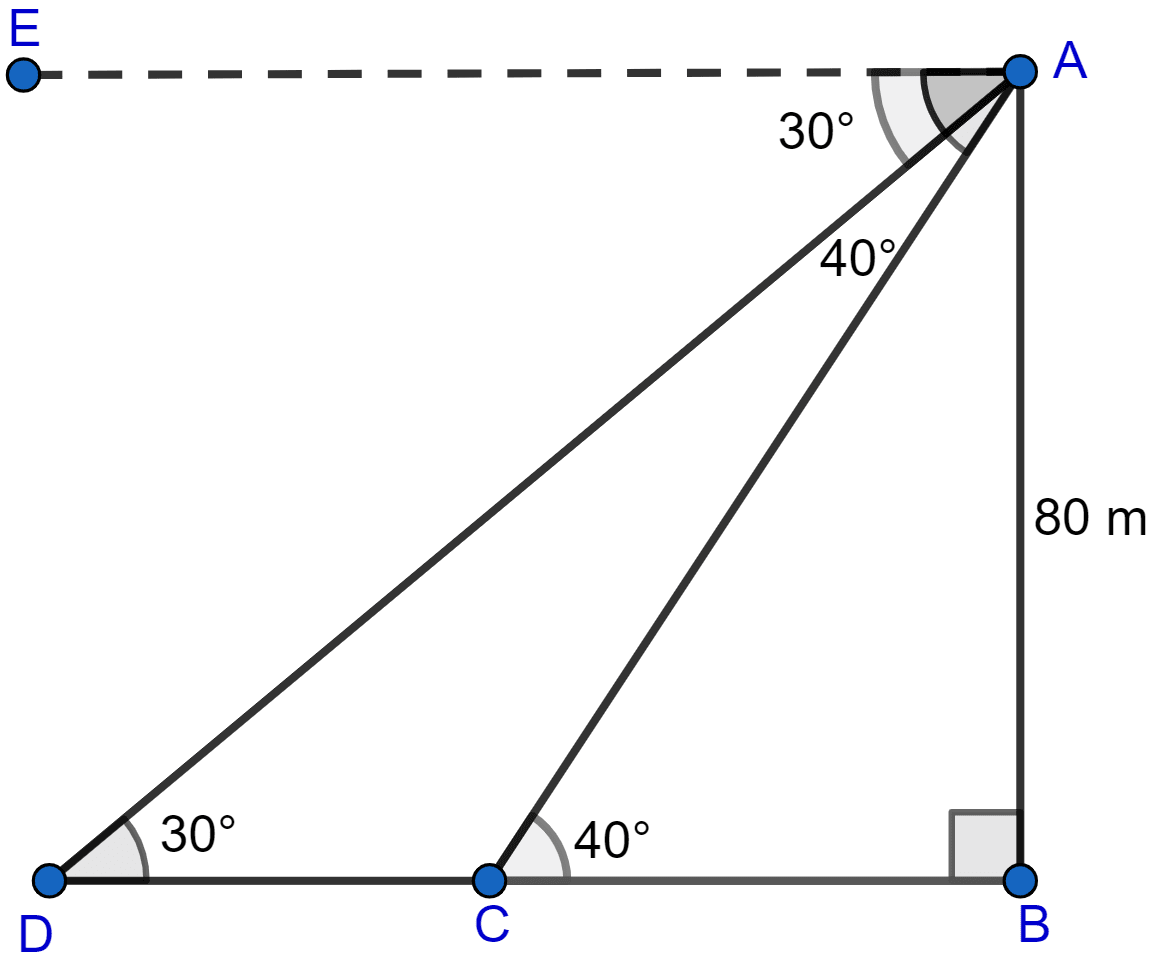
From figure,
∠ADB = ∠EAD = 30° (Alternate angles are equal)
∠ACB = ∠EAC = 40° (Alternate angles are equal)
Considering right angled △ADB, we get
Considering right angled △ACB, we get
Distance between two ships (DC) = DB - BC = 138.56 - 95.34 = 43.22 meters.
Rounding off to nearest meter DC = 43 meters.
Hence, the distance between two ships is 43 meters.
Answered By
23 Likes
Related Questions
In the adjoining figure, not drawn to the scale, AB is a tower and two objects C and D are located on the ground, on the same side of AB. When observed from the top A of the tower, their angles of depression are 45° and 60°. Find the distance between the two objects, if the height of the tower is 300 m. Give your answer to the nearest meter.

The horizontal distance between two towers is 140 m. The angle of elevation of the top of the first tower, when seen from the top of the second tower is 30°. If the height of the second tower is 60 m, find the height of the first tower.
The angle of elevation of a pillar from a point A on the ground is 45° and from a point B diametrically opposite to A and on the other side of the pillar is 60°. Find the height of the pillar, given that the distance between A and B is 15 m.
From two points A and B on the same side of a building, the angles of elevation of the top of the building are 30° and 60° respectively. If the height of the building is 10 m, find the distance between A and B correct to two decimal places.