Mathematics
A model of a ship is made to a scale of 1 : 250. Calculate :
(i) the length of the ship, if the length of model is 1.6 m.
(ii) the area of the deck of the ship, if the area of the deck of model is 2.4 m2.
(iii) the volume of the model, if the volume of the ship is 1 km3.
Similarity
19 Likes
Answer
(i) Since, the model of the ship is made to the scale of 1 : 250.
∴ K (Scale factor) = 250.
Actual length of the ship = k × (the length of model) = 250 × 1.6 = 400 m.
Hence, the length of the ship is 400 m.
(ii) Area of the deck of the ship = k2 × (Area of the deck of the model)
= (250)2 x 2.4
= 250 x 250 x 2.4
= 1,50,000 m2
Hence, the area of the deck of the ship is 1,50,000 m2.
(iii) Volume of the ship = k3 × (the volume of the model)
Given, volume of ship = 1 km3 = (1000)3 m3
Let the volume of the model be x m3.
Hence, the volume of the model of the ship is 64 m3.
Answered By
13 Likes
Related Questions
In the adjoining figure, D is a point on BC such that ∠ABD = ∠CAD. If AB = 5 cm, AC = 3 cm and AD = 4 cm, find
(i) BC
(ii) DC
(iii) area of △ACD : area of △BCA

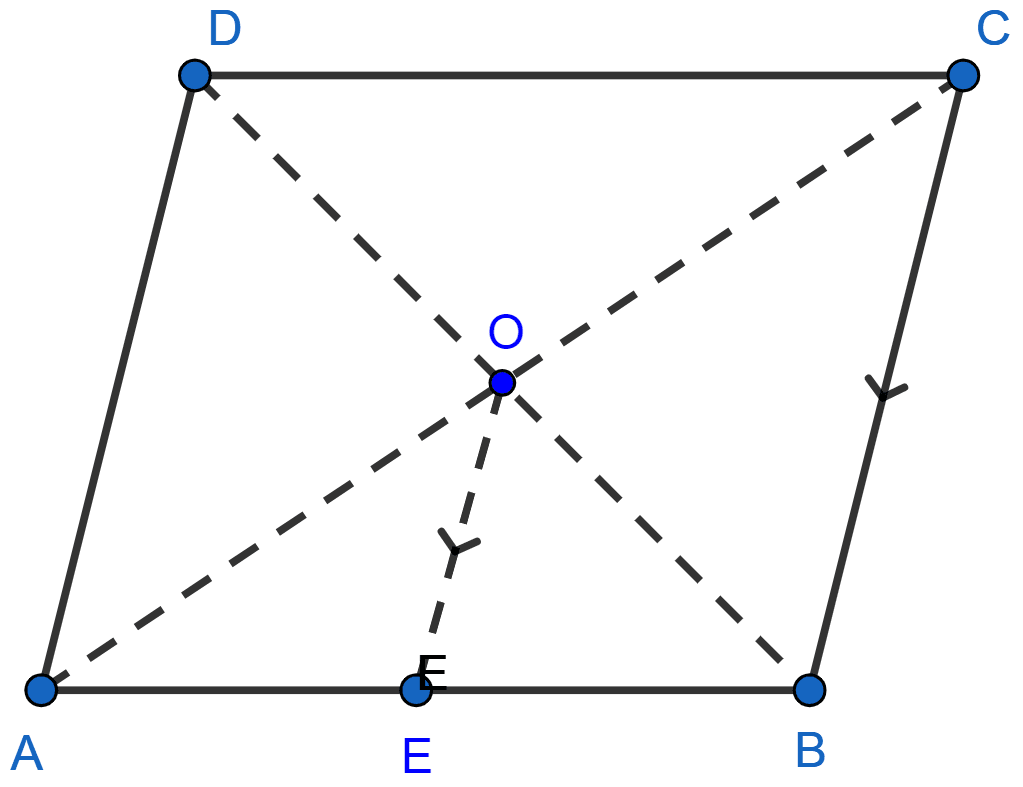
In the adjoining figure, the diagonals of a parallelogram intersect at O. OE is drawn parallel to CB to meet AB at E, find area of △AOE : area of ||gm ABCD.
In the adjoining figure, ABCD is a trapezium in which AB || DC. If 2AB = 3DC, find the ratio of the areas of △AOB and △COD.

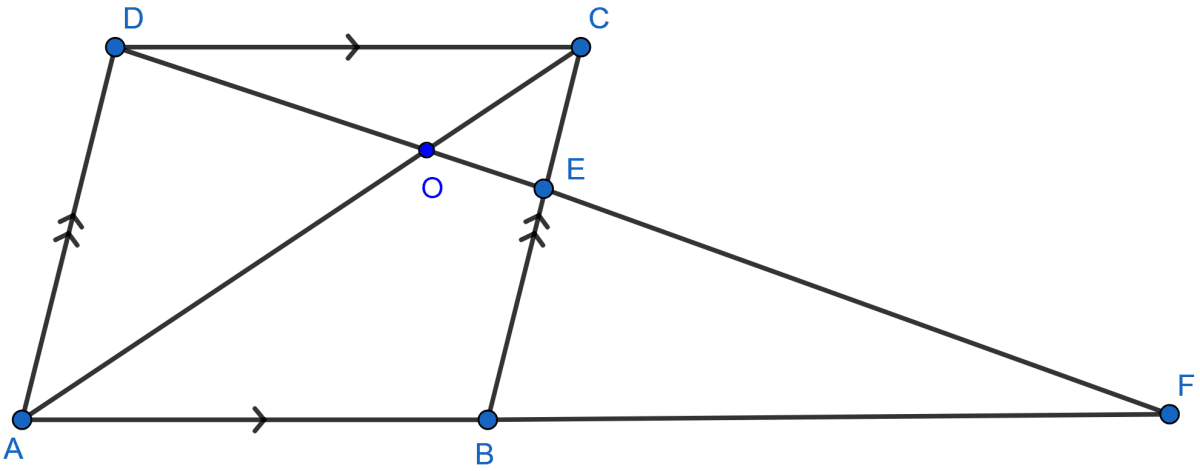
In the adjoining figure, ABCD is a parallelogram. E is mid-point of BC. DE meets the diagonal AC at O and meet AB (produced) at F. Prove that
(i) DO : OE = 2 : 1
(ii) area of △OEC : area of △OAD = 1 : 4