KnowledgeBoat Logo

Mathematics

A hemispherical depression is cut out from one face of a cubical wooden block such that the diameter l of the hemisphere is equal to the edge of the cube. Determine the surface area of the remaining solid.

Mensuration

7 Likes

Answer

Given,

Diameter of hemispherical depression = ll units.

Radius of hemispherical vessel = l2\dfrac{l}{2} units.

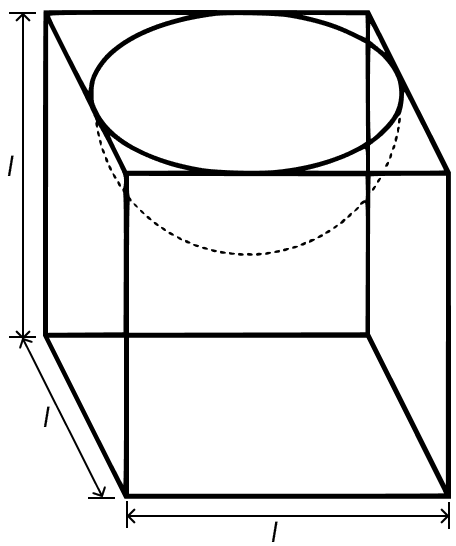
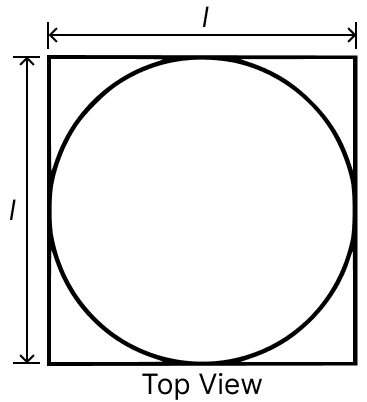
A hemispherical depression is cut out from one face of a cubical wooden block such that the diameter l of the hemisphere is equal to the edge of the cube. Determine the surface area of the remaining solid. NCERT Class 10 Mathematics CBSE Solutions.
A hemispherical depression is cut out from one face of a cubical wooden block such that the diameter l of the hemisphere is equal to the edge of the cube. Determine the surface area of the remaining solid. NCERT Class 10 Mathematics CBSE Solutions.

From figure,

Surface area of remaining solid = Surface area of cube + Curved surface area of hemisphere - Area of base of hemisphere

= 6(side)2 + 2πr2 - πr2

= 6(side)2 + πr2

= 6l2 + π ×(l2)2\times \Big(\dfrac{l}{2}\Big)^2

= 6l2 + l24π\dfrac{l^2}{4}π

= 24l2+l2π4\dfrac{24l^2 + l^2π}{4}

= l24(24+π)\dfrac{l^2}{4}(24 + π).

Hence, surface area of the remaining solid = l24(24+π)\dfrac{l^2}{4}(24 + π).

Answered By

2 Likes

Related Questions