Physics
A battery of e.m.f. 12 V and internal resistance 2 Ω is connected with two resistors A and B of resistance 4 Ω and 6 Ω respectively joined in series.
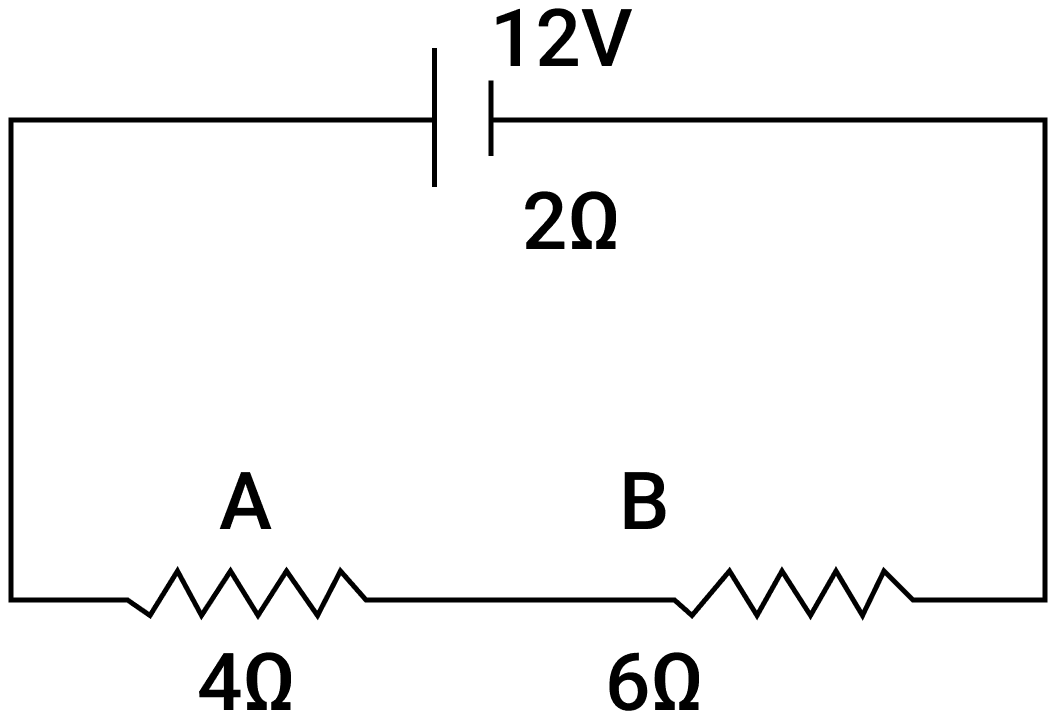
Find:
(i) Current in the circuit.
(ii) The terminal voltage of the cell.
(iii) The potential difference across 6 Ω Resistor.
(iv) Electrical energy spent per minute in 4 Ω Resistor.
Current Electricity
ICSE 2016
8 Likes
Answer
Given,
RA = 4 Ω
RB = 6 Ω
e.m.f. = 12 V
r = 2 Ω
(i) Two resistors ( 4 Ω and 6 Ω) are connected in series, so Rs = 4 + 6 = 10 Ω
From relation,
I =
Substituting the values we get,
(ii) Terminal voltage of cell V = ε - Ir
Substituting the values we get,
(iii) P.d. across 6 Ω resistor = I R6Ω
Substituting the values we get,
V6Ω = 1 x 6 = 6 V
(iv) Electrical energy spent per minute (60 s) in 4 Ω Resistor = I2RAt = 1 x 6 = 6 V
Answered By
6 Likes
Related Questions
(i) Which particles are responsible for current in conductors?
(ii) To which wire of a cable in a power circuit should the metal case of a geyser be connected?
(iii) To which wire should the fuse be connected?
(i) Name the transformer used in the power transmitting station of a power plant.
(ii) What type of current is transmitted from the power station?
(iii) At what voltage is this current available to our household?
Arrange α, β and γ rays in ascending order with respect to their
(i) Penetrating power.
(ii) Ionising power.
(iii) Biological effect.
(i) In a cathode ray tube what is the function of anode?
(ii) State the energy conversion taking place in a cathode ray tube.
(iii) Write one use of cathode ray tube.