KnowledgeBoat Logo

Mathematics

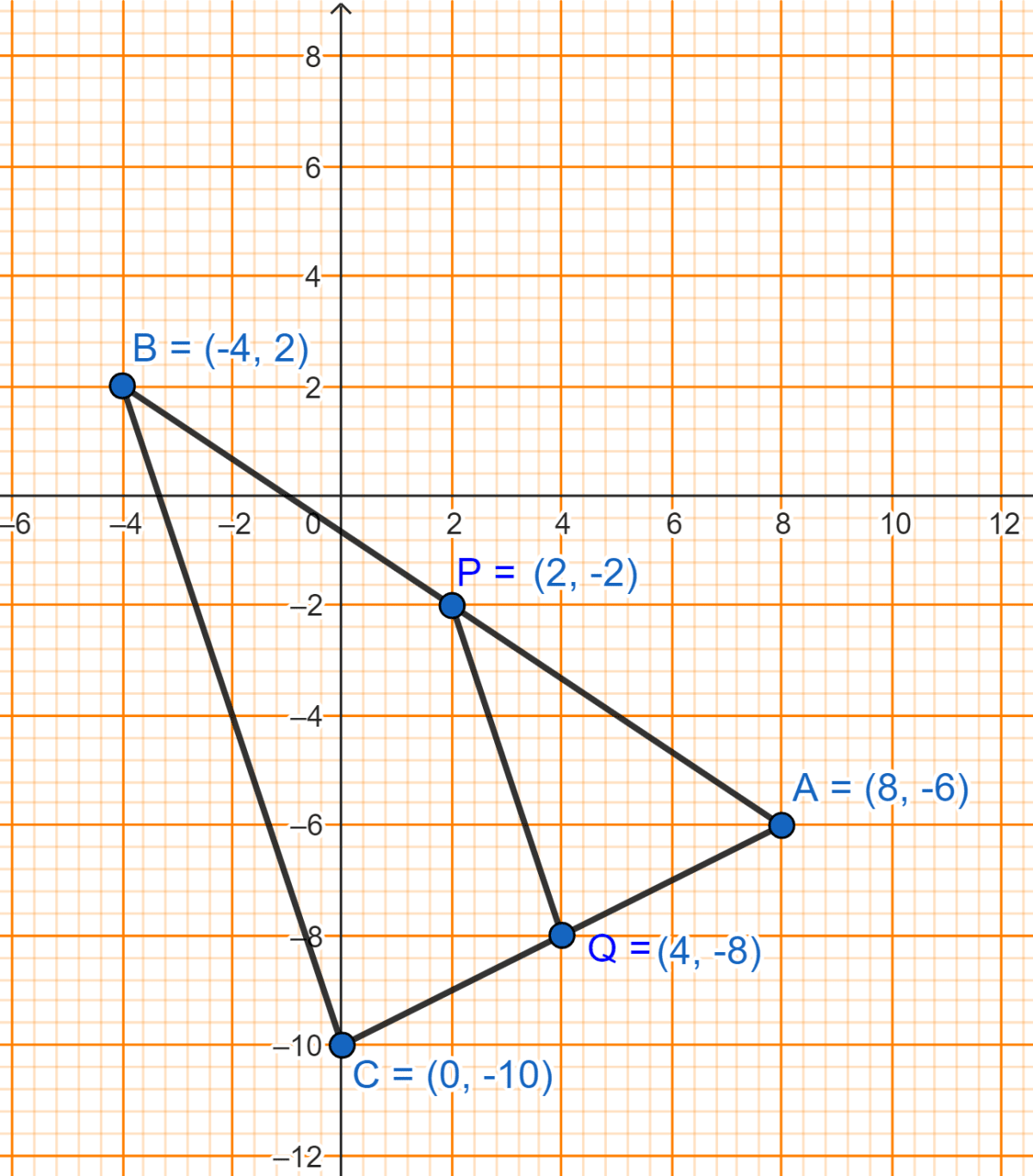
A (8, -6), B (-4, 2) and C (0, -10) are vertices of a triangle ABC. If P is the mid-point of AB and Q is the mid-point of AC, use co-ordinate geometry to show that PQ is parallel to BC. Give a special name of quadrilateral PBCQ.

Straight Line Eq

1 Like

Answer

ΔABC with vertices A (8, -6), B (-4, 2) and C (0, -10) is shown below:

A (8, -6), B (-4, 2) and C (0, -10) are vertices of a triangle ABC. If P is the mid-point of AB and Q is the mid-point of AC, use co-ordinate geometry to show that PQ is parallel to BC. Give a special name of quadrilateral PBCQ. Equation of a Line, Concise Mathematics Solutions ICSE Class 10.

By Mid-point formula,

Mid-point = (x1+x22,y1+y22)\Big(\dfrac{x1 + x2}{2}, \dfrac{y1 + y2}{2}\Big)

Co-ordinates of P = [8+(−4)2,−6+22]=(42,−42)=(2,−2).\Big[\dfrac{8 + (-4)}{2}, \dfrac{-6 + 2}{2}\Big] = \Big(\dfrac{4}{2}, \dfrac{-4}{2}\Big) = (2, -2).

Co-ordinates of Q = [8+02,−6+(−10)2]=(82,−162)\Big[\dfrac{8 + 0}{2}, \dfrac{-6 + (-10)}{2}\Big] = \Big(\dfrac{8}{2}, \dfrac{-16}{2}\Big) = (4, -8).

By formula,

Slope = y2−y1x2−x1\dfrac{y2 - y1}{x2 - x1}

Substituting values we get,

Slope of PQ = −8−(−2)4−2=−62\dfrac{-8 - (-2)}{4 - 2} = \dfrac{-6}{2} = -3.

Slope of BC = −10−20−(−4)=−124\dfrac{-10 - 2}{0 - (-4)} = \dfrac{-12}{4} = -3.

Since, slope of PQ = slope of BC.

∴ PQ || BC.

From figure,

PBCQ is a trapezium.

Answered By

1 Like

Related Questions