The following bill shows the GST rate and the marked price of items :
| S.No. | Item | Marked price (₹) | Quantity | Rate of GST |
|---|---|---|---|---|
| 1 | Wheat Flour (unpacked) | 35.00 | 5 kg | x% |
| 2 | Basmati Rice (Branded & packed) | 180.00 | 5 kg | 5% |
| 3 | Surf Excel Quick Wash Detergent | 220.00 | y kg | 18% |
Find :
(a) the value of x if the total GST on wheat flour and basmati rice is ₹ 45.
(b) the value of y, if CGST paid for detergent powder is ₹ 39.60
(c) total amount to be paid (including GST) for the above bill.
Answer
(a) Given, total GST on wheat flour and basmati rice is ₹ 45.
Total price of wheat flour = 35 × 5 = ₹ 175.
Total price of basmati rice = 180 × 5 = ₹ 900.
Given,
Total GST on wheat flour and basmati rice is ₹ 45.
Hence, x = 0%.
(b) From table,
Total price of surf excel detergent = 220 × y = ₹ 220y.
GST on detergent powder = 18%
CGST = = 9%.
Given,
CGST paid for detergent powder is ₹ 39.60
Hence, y = 2 kg.
(c) For wheat flour,
Total price = 35 × 5 = ₹ 175
GST = 0%
So, total price = ₹ 175.
For basmati rice,
Total price = 180 × 5 = ₹ 900
GST = 5%
So, total price = ₹ 900 + = ₹ 900 + ₹ 45 = ₹ 945.
For detergent powder,
Total price = 220 × 2 = ₹ 440
GST = 18%
So, total price = ₹ 440 + = ₹ 440 + ₹ 79.20 = ₹ 519.20
Total bill = ₹ 175 + ₹ 945 + ₹ 519.20 = ₹ 1639.20
Hence, total bill = ₹ 1639.20
Amit deposited ₹ 600 per month in a recurring deposit account. The bank pays a simple interest of 12% p.a. Calculate the:
(a) number of monthly installments Amit deposits to get a maturity amount of ₹ 11826?
(b) total interest paid by the bank.
(c) total amount deposited by him.
Answer
(a) Let money be deposited for n months.
By formula,
M.V. = P × n +
Substituting values we get :
Since, no. of months cannot be negative.
∴ n = 18.
Hence, number of monthly installments = 18.
(b) By formula,
Interest =
Substituting values we get :
Hence, total interest paid = ₹ 1026.
(c) Total amount deposited by Amit = P × n
= ₹ 600 × 18
= ₹ 10800.
Hence, total amount deposited by Amit = ₹ 10800.
Aman has 500, ₹ 100 shares of a company quoted at ₹ 120, paying a 10% dividend. When the share price rises to ₹ 200 each, he sells all his shares. He invests half of the sale proceeds in ₹ 10, 12% shares at ₹ 25, and the remaining sale proceeds in ₹ 400, 9% shares at ₹ 500.
Find his:
(a) sales proceeds.
(b) investment in ₹ 10, 12% shares at ₹ 25.
(c) original income.
(d) change in income.
Answer
(a) No. of shares Aman sells = 500
Aman sells the share when they rise to ₹ 200.
Sale proceeds = 500 × ₹ 200 = ₹ 1,00,000.
Hence, sale proceeds = ₹ 1,00,000.
(b) Given,
Aman invests half of the sale proceeds in ₹ 10, 12% shares at ₹ 25.
∴ Investment = = ₹ 50,000.
Hence, investment in ₹ 10, 12% shares at ₹ 25 = ₹ 50,000.
(c) By formula,
Income = No. of shares × × Nominal value of share
= 500 × × 100
= ₹ 5,000.
Hence, original income = ₹ 5,000.
(d) Aman invests ₹ 50,000 in each of the new shares.
For 1st share :
N.V. = ₹ 10
Dividend = 12%
M.V. = ₹ 25
No. of shares bought = = 2000.
Income = No. of shares × × Nominal value of share
= 2000 ×
= ₹ 2400.
For 2nd share :
N.V. = ₹ 400
Dividend = 9%
M.V. = ₹ 500
No. of shares bought = = 100.
Income = No. of shares × × Nominal value of share
= 100 ×
= ₹ 3,600.
New income = ₹ 3,600 + ₹ 2,400 = ₹ 6000
Change in income = New income - Original income = ₹ 6,000 - ₹ 5,000 = ₹ 1,000.
Hence, change in income = ₹ 1,000 (increase).
Solve the following inequation.
, x ∈ R
(a) Write the solution set.
(b) Represent the solution on the number line.
Answer
Given,
Solving L.H.S. of the equation,
Solving R.H.S. of the equation,
From equation (1) and (2), we get :
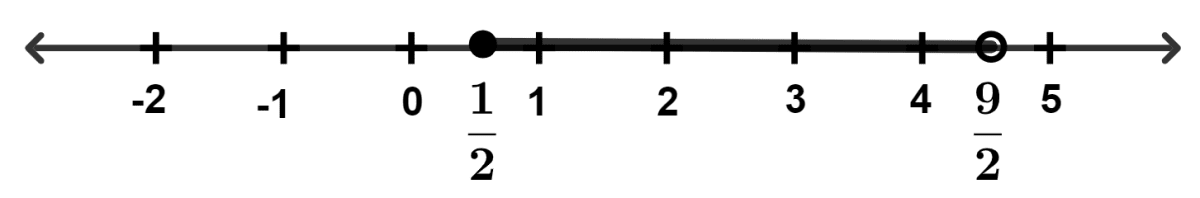
Hence, solution set = {}
Determine whether the following quadratic equation has real roots.
5𝑥2 − 9𝑥 + 4 = 0
(a) Give reasons for your answer.
(b) If the equation has real roots, identify them.
Answer
(a) Given, equation : 5𝑥2 − 9𝑥 + 4 = 0
Comparing above equation with ax2 + bx + c = 0, we get :
a = 5, b = -9 and c = 4.
Discriminant (D) = b2 - 4ac = (-9)2 - 4 × 5 × 4 = 81 - 80 = 1.
Since, D > 0 and a perfect square.
Hence, equation 5𝑥2 − 9𝑥 + 4 = 0 has real roots.
(b) Solving,
⇒ 5𝑥2 − 9𝑥 + 4 = 0
⇒ 5x2 - 5x - 4x + 4 = 0
⇒ 5x(x - 1) - 4(x - 1) = 0
⇒ (5x - 4)(x - 1) = 0
⇒ 5x - 4 = 0 or x - 1 = 0
⇒ 5x = 4 or x = 1
⇒ x = or x = 1.
Hence, roots of the equation are 1 and .
The profit in rupees in a local restaurant and the number of customers who visited the restaurant are tabulated below for each week for one month.
| Week number | Week 1 | Week 2 | Week 3 | Week 4 |
|---|---|---|---|---|
| Number of customers | 1400 | 5600 | x | 3212 |
| Profit in ₹ | 28000 | 112000 | 32140 | y |
Find :
(a) if the number of customers and profit per week in continued proportion or not? Justify your answer.
(b) the value of x and y.
Answer
(a) Since,
.
Hence, number of customers and profit per week are in proportion but not in continued proportion.
(b) As,
Number of customers and profit per week are in proportion.
and
Hence, x = 1607 and y = 64240.
Given, 9𝑥2 - 4 is a factor of 9𝑥3 - m𝑥2 - n𝑥 + 8 :
(a) find the value of m and n using the remainder and factor theorem.
(b) factorise the given polynomial completely.
Answer
(a) Simplifying 9𝑥2 - 4, we get :
⇒ 9𝑥2 - 4
⇒ (3𝑥)2 - 22
⇒ (3𝑥 + 2)(3𝑥 - 2).
We know that,
If (x - a) is a factor of f(x), then f(a) = 0.
Given,
9𝑥2 - 4 is a factor of 9𝑥3 - m𝑥2 - n𝑥 + 8.
∴ (3𝑥 + 2) and (3𝑥 - 2) are the factors of 9𝑥3 - m𝑥2 - n𝑥 + 8.
⇒ 3𝑥 + 2 = 0
⇒ 3𝑥 = -2
⇒ 𝑥 =
Substituting x = in 9𝑥3 - mx2 - nx + 8, we get remainder = 0.
⇒ 3𝑥 - 2 = 0
⇒ 3𝑥 = 2
⇒ 𝑥 =
Substituting 𝑥 = in 9𝑥3 - m𝑥2 - n𝑥 + 8, we get remainder = 0.
Adding equation (1) and (2), we get :
⇒ 3n - 2m + 2m + 3n = -24 + 48
⇒ 6n = 24
⇒ n =
⇒ n = 4.
Substituting value of n in equation (1), we get :
⇒ 3(4) - 2m = -24
⇒ 12 - 2m = -24
⇒ -2m = -24 - 12
⇒ -2m = -36
⇒ m = = 18.
Hence, m = 18 and n = 4.
(b) Substituting value of m and n in 9𝑥3 - m𝑥2 - n𝑥 + 8, we get :
9𝑥3 - 18𝑥2 - 4𝑥 + 8
Dividing 9𝑥3 - 18𝑥2 - 4𝑥 + 8 by 9𝑥2 - 4, we get :
∴ 9𝑥3 - 18𝑥2 - 4𝑥 + 8 = (9𝑥2 - 4)(𝑥 - 2)
= (3𝑥 + 2)(3𝑥 - 2)(𝑥 - 2).
Hence, factors are (3𝑥 + 2), (3𝑥 - 2) and (𝑥 - 2).
The marks scored by 100 students are given below:
| Marks scored | No. of students |
|---|---|
| 0-10 | 4 |
| 10-20 | 5 |
| 20-30 | 9 |
| 30-40 | 7 |
| 40-50 | 13 |
| 50-60 | 12 |
| 60-70 | 15 |
| 70-80 | 11 |
| 80-90 | 14 |
| 90-100 | 10 |
A student in the class is selected at random. Find the probability that the student has scored:
(a) less than 20
(b) below 60 but 30 or more
(c) more than or equal to 70
(d) above 89.
Answer
| Marks scored | No. of students | Cumulative frequency |
|---|---|---|
| 0-10 | 4 | 4 |
| 10-20 | 5 | 9 |
| 20-30 | 9 | 18 |
| 30-40 | 7 | 25 |
| 40-50 | 13 | 38 |
| 50-60 | 12 | 50 |
| 60-70 | 15 | 65 |
| 70-80 | 11 | 76 |
| 80-90 | 14 | 90 |
| 90-100 | 10 | 100 |
(a) By formula,
Probability that student has scored less than 20
= .
Hence, probability that the student has scored less than 20 = .
(b) From table,
No. of students who scored less than 60 = 50
No. of students who scored less than 30 = 18
∴ No. of students who score below 60 but 30 or more = 50 - 18 = 32.
Probability that student has scored below 60 but 30 or more = .
Hence, probability that the student has scored below 60 but 30 or more = .
(c) From table,
No. of students who scored less than 70 = 65
Total no. of students = 100
∴ No. of students who score more than or equal to 70 = 100 - 65 = 35.
Probability that student has scored more than or equal to 70 = .
Hence, probability that the student has scored more than or equal to 70 = .
(d) No. of students those who have scored more than 89 = No. of students who has scored between 90-100 = 10.
Probability that student has scored more than 89 = .
Hence, probability that the student has scored more than 89 = .
Given, matrix A = such that AB is a null matrix. Find :
(a) order of the null matrix
(b) possible values of x and y.
Answer
Given,
AB is a null matrix.
Let AB = X, where X is a null matrix of order a × b.
⇒ AB = X
⇒ A2 × 2 × B2 × 1 = Xa × b
We know that,
The resultant matrix has no. of rows equal to the rows in the first matrix and no. of columns equal to the no. of columns in the second matrix.
∴ a = 2 and b = 1.
From equation (1) :
⇒ x2 + x - 2 = 0
⇒ x2 + 2x - x - 2 = 0
⇒ x(x + 2) - 1(x + 2) = 0
⇒ (x - 1)(x + 2) = 0
⇒ x - 1 = 0 or x + 2 = 0
⇒ x = 1 or x = -2.
From equation (1) :
⇒ xy + 2x - 4 = 0
Substituting x = 1, we get :
⇒ 1.y + 2.1 - 4 = 0
⇒ y + 2 - 4 = 0
⇒ y - 2 = 0
⇒ y = 2.
Substituting x = -2, we get :
⇒ (-2).y + 2.(-2) - 4 = 0
⇒ -2y - 4 - 4 = 0
⇒ -2y - 8 = 0
⇒ -2y = 8
⇒ y = = -4.
Hence, x = 1, y = 2 or x = -2, y = -4.
The sum of a certain number of terms of the Arithmetic Progression (A.P.) 20, 17, 14, ....... is 65. Find the:
(a) number of terms.
(b) last term.
Answer
(a) Let no. of terms be n.
By formula,
Sum of A.P. =
Substituting values we get :
Since, no. of terms cannot be in fraction.
∴ n = 10.
Hence, no. of terms = 10.
(b) By formula,
Last term (l) = a + (n - 1)d
= 20 + (10 - 1) × (-3)
= 20 + 9 × (-3)
= 20 - 27
= -7.
Hence, last term = -7.
(a) Point P(2, -3) on reflection becomes P'(2, 3). Name the line of reflection (say L1).
(b) Point P' is reflected to P'' along the line (𝐿2), which is perpendicular to the line 𝐿1 and passes through the point, which is invariant along both axes. Write the coordinates of P''.
(c) Name and write the coordinates of the point of intersection of the lines 𝐿1 and 𝐿2.
(d) Point P is reflected to P''' on reflection through the point named in the answer of part I of this question. Write the coordinates of P'''. Comment on the location of the points P'' and P'''.
Answer
(a) P(2, -3) ⇒ P'(2, 3)
Since, sign of y-coordinate changes.
∴ L1 = x-axis
Hence, point P becomes P' on reflection in x-axis.
(b) Origin remains invariant on reflection along both the axes.
L2 is perpendicular to L1.
It means L2 is perpendicular to x-axis and passes through (0, 0).
∴ L2 is y-axis.
P'(2, 3) on reflection in y-axis becomes P''(-2, 3).
Hence, coordinates of P'' = (-2, 3).
(c) x-axis and y-axis intersect at origin.
Hence, coordinates of intersection of lines L1 and L2 is (0, 0).
(d) P(2, -3) on reflection in origin becomes P'''(-2, 3).
Since, P'' and P''' have similar co-ordinates.
Hence, P'' and P''' are coincident points.
In the given figure, if the line segment AB is intercepted by the y-axis and x-axis at C and D, respectively, such that AC : AD = 1 : 4 and D is the midpoint of CB. Find the coordinates of D, C and B.
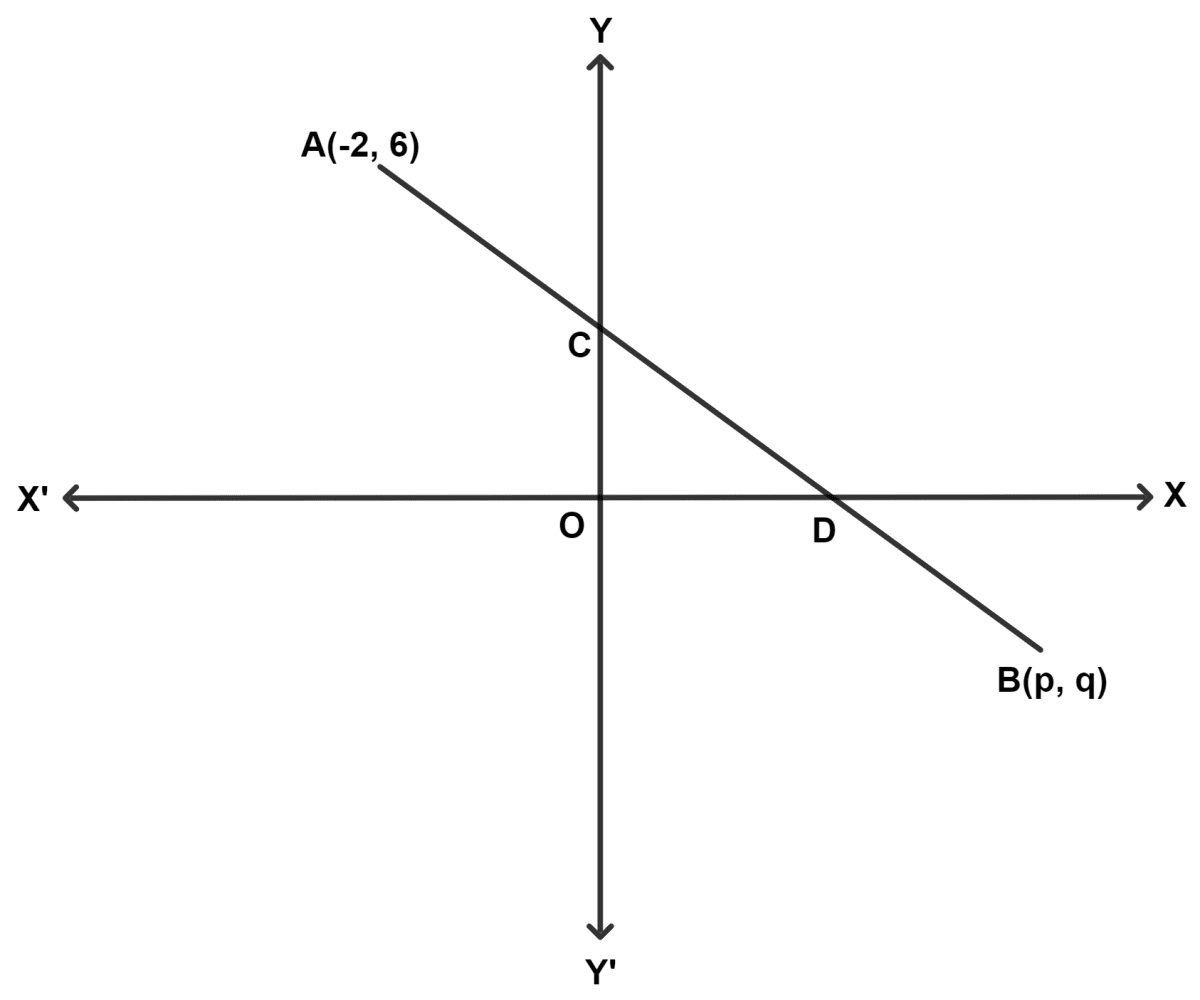
Answer
Let coordinates of C be (0, b) and D be (a, 0).
Given,
AC : AD = 1 : 4
Let AC = x and AD = 4x.
From figure,
⇒ AD = AC + CD
⇒ 4x = x + CD
⇒ CD = 4x - x = 3x.
AC : CD = 1 : 3.
By section formula,
C = (0 , b) = and D = (6, 0).
Given, D is the mid-point of CB.
Hence, coordinates of B = and D = (6, 0).
Find the equation of the straight line perpendicular to the line x + 2y = 4, which cuts an intercept of 2 units from the positive y-axis. Hence, find the intersection point of the two lines.
Answer
Given,
Equation : x + 2y = 4
⇒ 2y = -x + 4
⇒ y =
Comparing above equation with y = mx + c we get :
⇒ m =
Let slope of line perpendicular to line x + 2y = 4 be m1.
We know that,
Product of slope of perpendicular lines = -1.
Substituting values we get :
⇒ y = mx + c
⇒ y = 2x + 2.
Simultaneously solving equation :
⇒ x + 2y = 4 .........(1)
⇒ y = 2x + 2 .......(2)
Substituting value of y from equation (2) in (1), we get :
⇒ x + 2(2x + 2) = 4
⇒ x + 4x + 4 = 4
⇒ 5x = 4 - 4
⇒ 5x = 0
⇒ x = = 0.
Substituting value of x in equation (2), we get :
⇒ y = 2(0) + 2 = 2.
Hence, equation of required line is y = 2x + 2 and point of intersection = (0, 2).
While preparing a PowerPoint presentation, ∆ ABC is enlarged along the side BC to ∆ AB'C', as shown in the diagram, such that BC ∶ B'C' is 3 ∶ 5. Find :
(a) AB ∶ BB'
(b) length AB, if BB' = 4 cm.
(c) Is ∆ ABC ~ ∆ AB'C' ? Justify your answer.
(d) ar (∆ ABC) : ar (quad. BB'C'C).
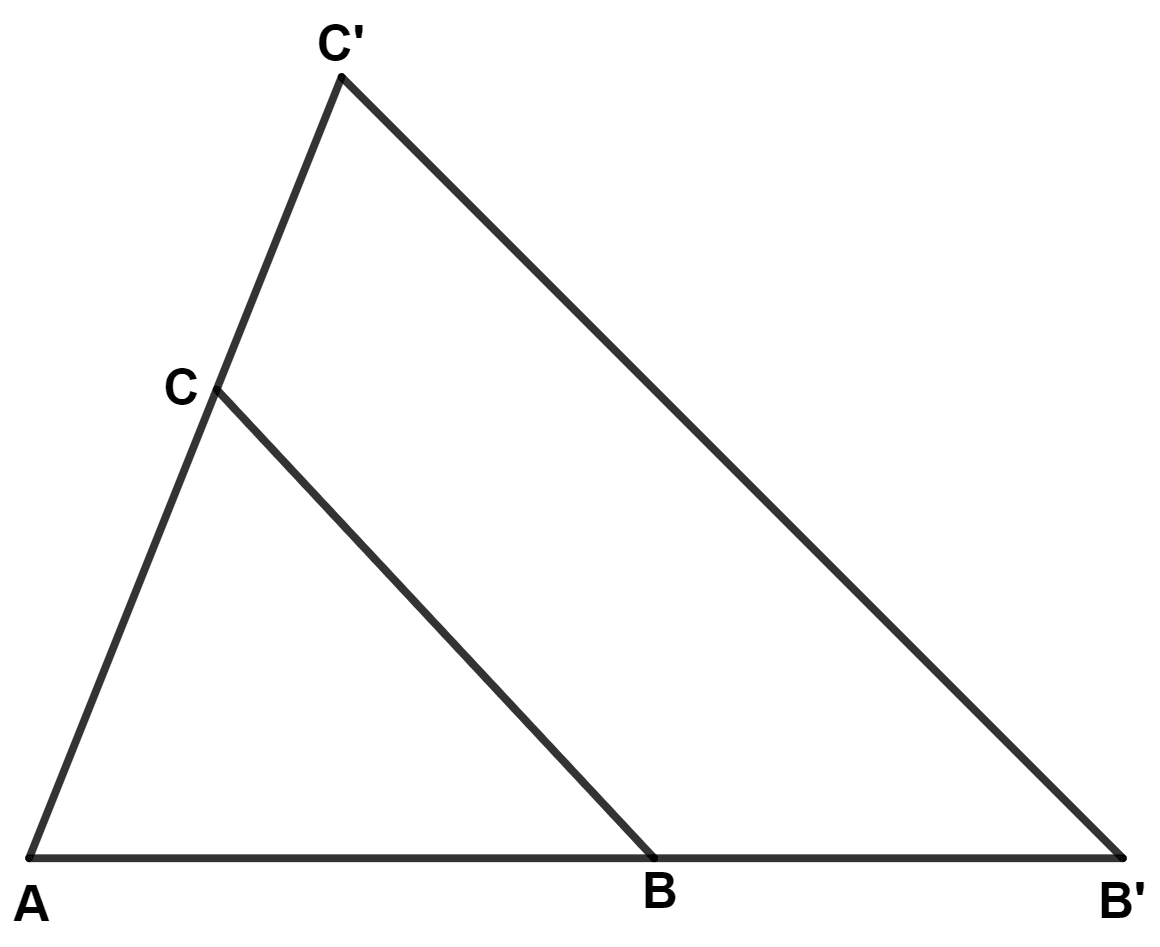
Answer
Since, ∆ ABC is enlarged along the side BC to ∆ AB'C'.
∴ ∆ ABC and ∆ AB'C' are similar triangles.
(a) We know that,
Ratio of corresponding sides of similar triangles are proportional.
Let AB = 3x and AB' = 5x
From figure,
⇒ AB' = AB + BB'
⇒ 5x = 3x + BB'
⇒ BB' = 5x - 3x = 2x.
⇒ AB : BB' = 3x : 2x = 3 : 2.
Hence, AB : BB' = 3 : 2.
(b) As,
Hence, AB = 6 cm.
(c) Since, ∆ ABC is enlarged along the side BC to ∆ AB'C'.
∴ BC || B'C'
In ∆ ABC and ∆ AB'C',
⇒ ∠BAC = ∠B'AC' (Common angle)
⇒ ∠ABC = ∠AB'C' (Corresponding angle are equal)
∴ ∆ ABC ~ ∆ AB'C' (By A.A. axiom).
Hence, proved that ∆ ABC ~ ∆ AB'C'.
(d) We know that,
The ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides.
Let area of ∆ ABC = 9a and area of ∆ AB'C' = 25a.
Area of quadrilateral BB'C'C = Area of ∆ AB'C' - Area of ∆ ABC = 25a - 9a = 16a.
∴ Area of ∆ ABC : Area of quadrilateral BB'C'C = 9a : 16a = 9 : 16.
Hence, area of ∆ ABC : area of quadrilateral BB'C'C = 9 : 16.
The approximate volume of a human eye is 6.5 cm3. The volume of a laboratory model (excluding base and stand) of the human eye is 1404 cm3.

(a) State whether the scale factor k is less than, equals to or greater than 1.
(b) Calculate the:
(i) value of k
(ii) diameter of the human eye if the radius of the model is 7.2 cm.
(iii) the external surface area of the human eye if the surface area of the model is 651.6 cm2.
Answer
(a) Scale factor is greater than 1 as volume of model is greater than the original eye.
(b)
(i) By formula,
Hence, scale factor (k) = 6.
(ii) By formula,
Diameter of human eye = 1.2 × 2 = 2.4 cm
Hence, diameter of human eye = 2.4 cm.
(iii) By formula,
Hence, surface area of human eye = 18.1 cm2.
In the adjoining diagram PQ, PR and ST are the tangents to the circle with centre O and radius 7 cm. Given OP = 25 cm. Find :
(a) length of ST
(b) value of ∠OPQ, i.e. θ
(c) ∠QUR, in nearest degree
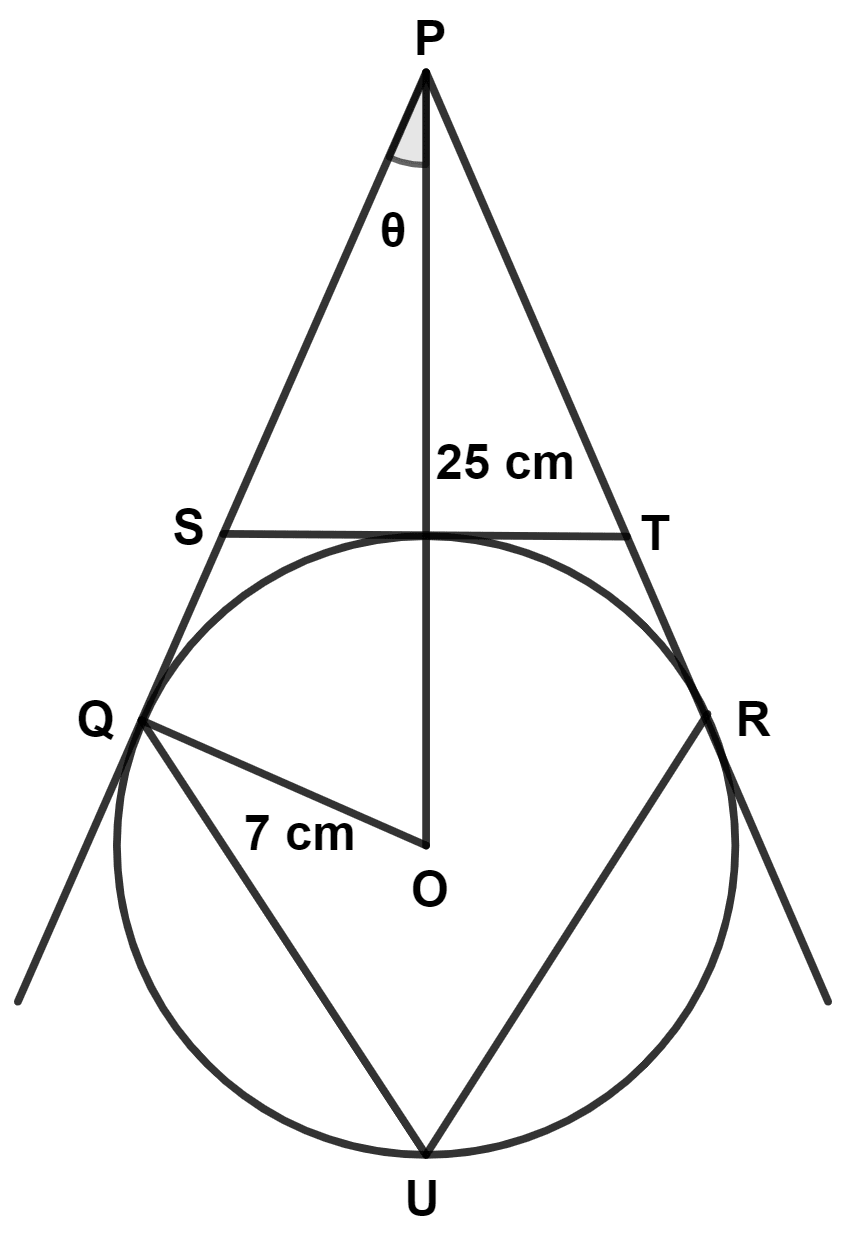
Answer
(a) We know that,
The radius of a circle and tangent are perpendicular at the point of contact.
∴ ∠PQO = 90°
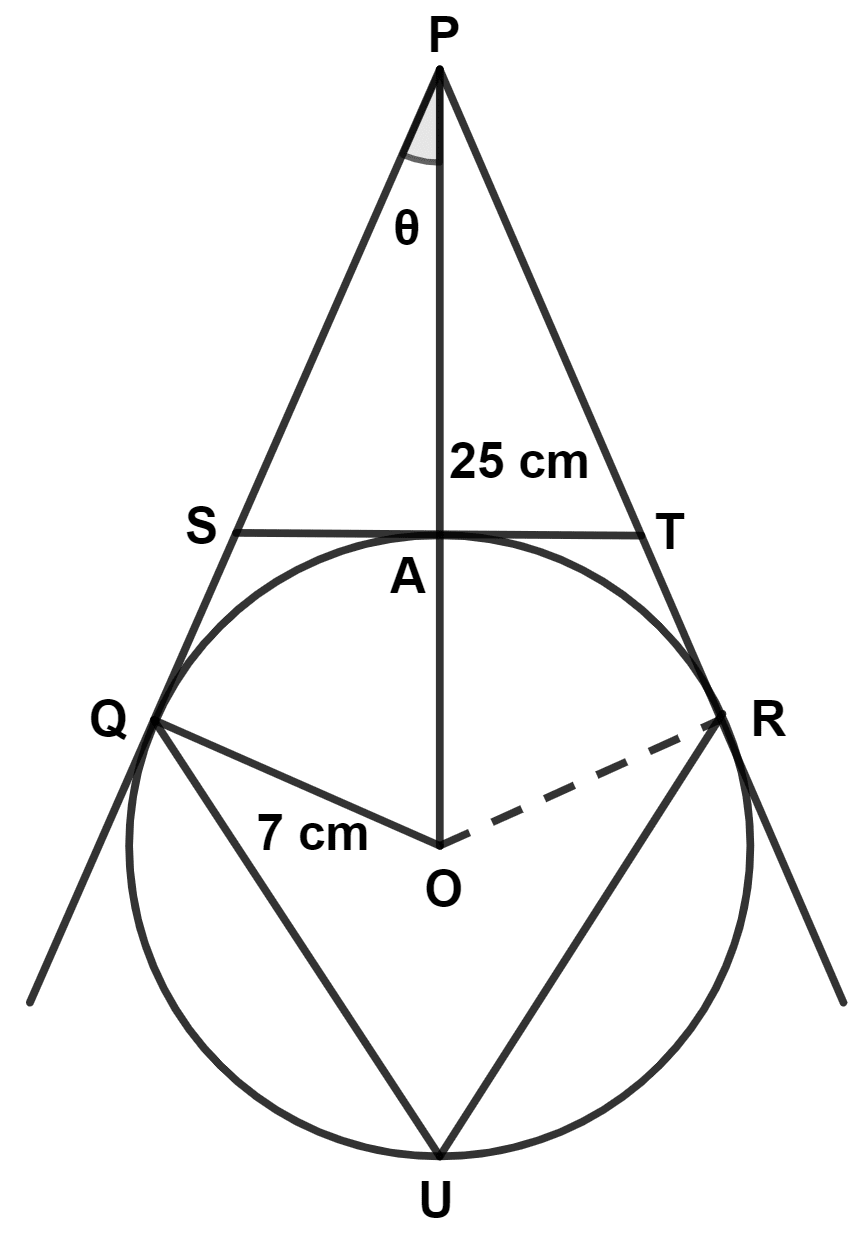
In right-angled triangle PQO,
⇒ PO2 = PQ2 + OQ2
⇒ 252 = PQ2 + 72
⇒ 625 = PQ2 + 49
⇒ PQ2 = 625 - 49
⇒ PQ2 = 576
⇒ PQ = = 24 cm.
In △ POQ,
⇒ tan θ =
⇒ tan θ = ...............(1)
From figure,
⇒ ∠PAS = 90°
⇒ PA = OP - OA = 25 - 7 = 18 cm.
⇒ tan θ =
⇒ tan θ = ...........(2)
From equation (1) and (2), we get :
From figure,
⇒ ST = 2 × AS = = 10.5 cm
Hence, ST = 10.5 cm.
(b) From equation (1),
⇒ tan θ =
⇒ tan θ = 0.292
⇒ tan θ = tan 16° 16'
⇒ θ = 16° 16'.
Hence, θ = 16° 16'.
(c) In △ POQ,
⇒ ∠POQ + ∠PQO + ∠QPO = 180°
⇒ ∠POQ + 90° + 16° 16' = 180°
⇒ ∠POQ + 106° 16' = 180°
⇒ ∠POQ = 180° - 106° 16' = 73° 44' = 74°.
We know that,
Tangent from an external point to the circle are equal in length.
∴ PQ = PR.
Also,
OR = OQ (Both equal to radius of circle)
In △ POQ and △ POR,
⇒ PQ = PR (Proved above)
⇒ OQ = OR (Radius of same circle)
⇒ PO = PO (Common side)
∴ △ POQ ≅ △ POR (By S.S.S. axiom)
We know that,
Corresponding parts of congruent triangles are equal.
∴ ∠POR = ∠POQ = 74°
From figure,
⇒ ∠QOR = ∠POR + ∠POQ = 74° + 74° = 148°.
We know that,
The angle subtended by an arc of a circle at its center is twice the angle it subtends anywhere on the circle's circumference.
⇒ ∠QOR = 2∠QUR
⇒ ∠QUR = = 74°.
Hence, ∠QUR = 74°.
Use ruler and compass to answer this question. Construct a triangle ABC where AB = 5.5 cm, BC = 4.5 cm and angle ABC = 135°. Construct the circumcircle to the triangle ABC. Measure and write down the length of AC.
Answer
Steps of construction :
Draw a line segment BC = 4.5 cm
Construct XB such that ∠XBC = 135°.
Cut AB = 5.5 cm from XB.
Join and measure AC.
Draw PQ and RS the perpendicular bisectors of BC and AB.
Mark point O the intersection of PQ and RS.
With O as center and radius as OA, draw a circle touching the vertices A, B and C.
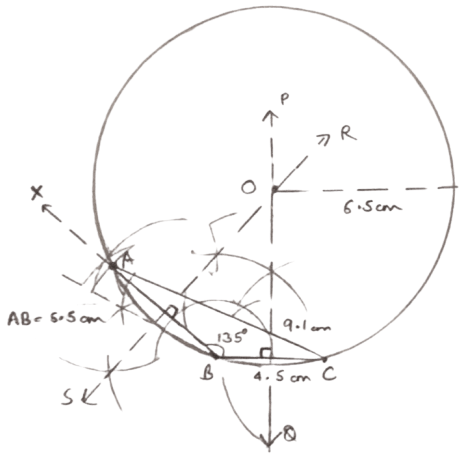
On measuring AC = 9.1 cm and radius = 6.5 cm.
The curved surface area of a right circular cone is half of another right circular cone. If the ratio of their slant heights is 2 : 1 and that of their volumes is 3 : 1, find ratio of their:
(a) radii
(b) heights
Answer
(a) Let radius of smaller and larger cone be r and R, respectively.
Let height of smaller and larger cone be h and H, respectively.
Let slant height of smaller and larger cone be l and L respectively.
Given,
Ratio of their slant heights is 2 : 1.
∴ l : L = 2 : 1.
Given,
Curved surface area of a right circular cone is half of the other right circular cone.
∴ πrl =
⇒ rl =
⇒
⇒
⇒ r : R = 1 : 4
Hence, ratio of the radii = 1 : 4.
(b) Let volume of cone with smaller curved surface area be v and that with larger curved surface area be V.
Given,
Volumes are in the ratio 3 : 1.
∴ v : V = 3 : 1
Hence, ratio of heights = 48 : 1.
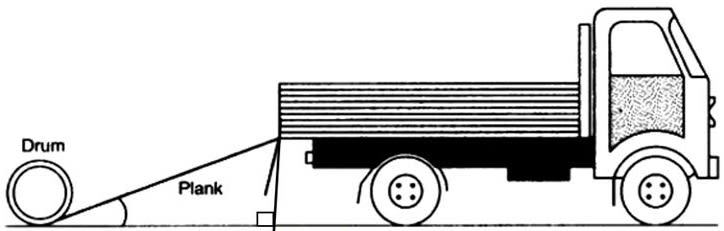
A cylindrical drum is unloaded from a truck by rolling it down along a wooden plank. The length of the plank is 10 m and it is making an angle of 10° with the horizontal ground. Find the height from which the cylindrical drum was rolled down. Give your answer correct to 3 significant figures.
Answer
Let AC be the plank and BC be the height from where the drum is rolled down.
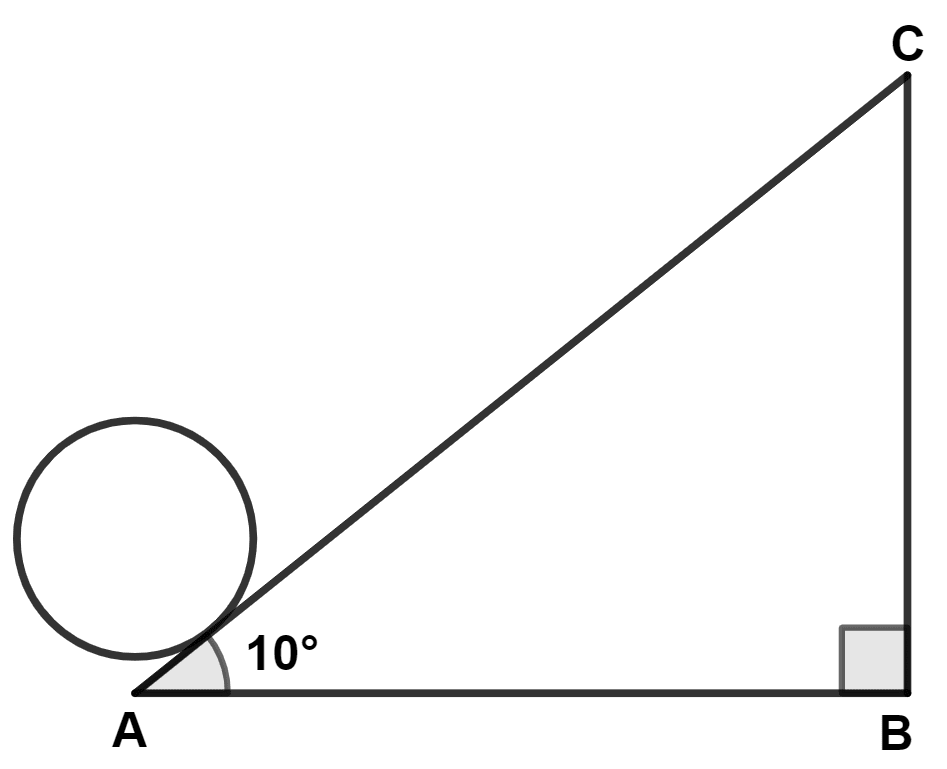
⇒ sin θ =
⇒ sin 10° =
⇒ 0.1736 =
⇒ BC = 1.736 ~ 1.74 m
Hence, height from which the cylindrical drum was rolled down = 1.74 m.
The data given below shows the marks of 12 students in a test, arranged in ascending order :
2, 3, 3, 3, 4, x, x + 2, 8, p, q, 8, 9.
If the given value of the median and mode is 6 and 8 respectively, then find the values of x, p, q.
Answer
By formula,
Median =
Substituting values we get :
Numbers :
2, 3, 3, 3, 4, 5, 7, 8, p, q, 8, 9.
Since, mode = 8, it means 8 occurs for the most times in the series.
Since, 3 occurs 3 times in the series,
∴ 8 must occur for atleast 4 times in order to be the mode.
∴ p = q = 8.
Hence, x = 5, p = 8 and q = 8.
Solve the linear inequation, write down the solution set and represent it on the real number line :
5(2 - 4x) > 18 - 16x > 22 - 20x, x ∈ R
Answer
Given,
5(2 - 4x) > 18 - 16x > 22 - 20x
Solving L.H.S. of the above equation :
⇒ 5(2 - 4x) > 18 - 16x
⇒ 10 - 20x > 18 - 16x
⇒ -16x + 20x < 10 - 18
⇒ 4x < -8
⇒ x <
⇒ x < -2 ...........(1)
Solving R.H.S. of the above equation :
⇒ 18 - 16x > 22 - 20x
⇒ 20x - 16x > 22 - 18
⇒ 4x > 4
⇒ x >
⇒ x > 1 ............(2)
From equation (1) and (2), we get :
Solution set : {x : x < -2 or x > 1, x ∈ R}
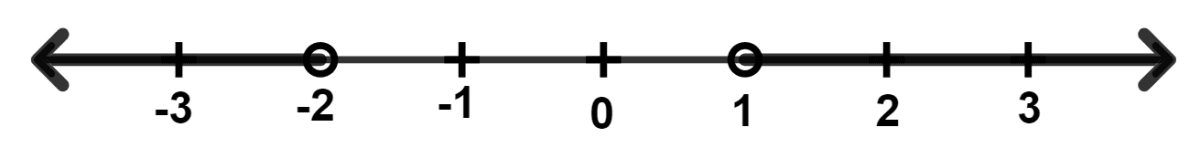
Hence, solution set = {x : x < -2 or x > 1, x ∈ R}.
If a polynomial x3 + 2x2 – ax + b leaves a remainder -6 when divided by x + 1 and the same polynomial has x - 2 as a factor, then find the values of a and b.
Answer
Given,
x3 + 2x2 – ax + b leaves a remainder -6 when divided by x + 1.
⇒ x + 1 = 0
⇒ x = -1.
Let f(x) = x3 + 2x2 – ax + b, then f(-1) = -6.
⇒ f(-1) = -6
⇒ (-1)3 + 2(-1)2 - a(-1) + b = -6
⇒ -1 + 2(1) + a + b = -6
⇒ -1 + 2 + a + b = -6
⇒ a + b + 1 = -6
⇒ a + b = -6 - 1
⇒ a + b = -7 ........(1)
Given ,
x - 2 is a factor of f(x).
⇒ x - 2 = 0
⇒ x = 2.
f(2) = 0
⇒ 23 + 2(2)2 – 2a + b = 0
⇒ 8 + 2(4) - 2a + b = 0
⇒ 8 + 8 - 2a + b = 0
⇒ 16 - 2a + b = 0
⇒ 2a - b = 16 ......(2)
Adding equation (1) and (2), we get :
⇒ a + b + 2a - b = -7 + 16
⇒ 3a = 9
⇒ a = = 3.
Substituting value of a = 3 in equation (1), we get :
⇒ 3 + b = -7
⇒ b = -7 - 3 = -10.
Hence, a = 3 and b = -10.
If A =
(a) Is the product AC possible? Justify your answer.
(b) Find the matrix X, such that X = AB + B2 − DC
Answer
(a) Order of matrix A = 2 × 2, Order of matrix C = 1 × 2
The product AC is not possible as the no. of columns in A is not equal to the no. of rows in C.
Hence, product AC is not possible.
(b) Given,
Hence, X =
In the given figure (not drawn to scale), BC is parallel to EF, CD is parallel to FG, AE : EB = 2 : 3, ∠BAD = 70°, ∠ACB = 105°, ∠ADC = 40° and AC is bisector of ∠BAD.
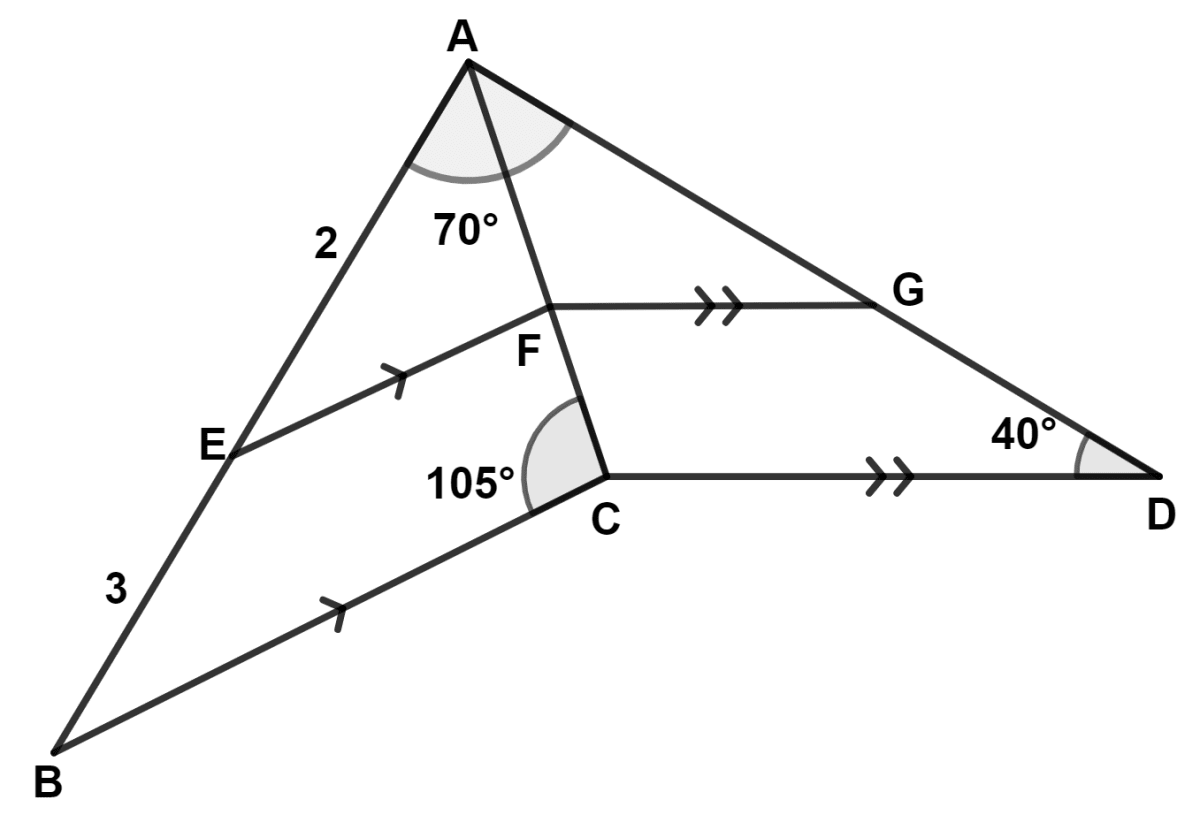
(a) Prove Δ AEF ~ Δ AGF
(b) Find :
(i) AG : AD
(ii) area of Δ ACB: area Δ ACD
(iii) area of quadrilateral ABCD: area of Δ ACB.
Answer
(a) In Δ AFE,
⇒ ∠AFE = ∠ACB = 105° (Corresponding angle are equal)
⇒ ∠EAF = = 35° (AC is the bisector of ∠BAD)
By angle sum property of triangle,
⇒ ∠AFE + ∠EAF + ∠AEF = 180°
⇒ 105° + 35° + ∠AEF = 180°
⇒ 140° + ∠AEF = 180°
⇒ ∠AEF = 180° - 140° = 40°.
∠AGF = ∠ADC = 40° (Corresponding angles are equal)
In Δ AGF,
⇒ ∠GAF = = 35° (AC is the bisector of ∠BAD)
In Δ AEF and Δ AGF,
⇒ ∠EAF = ∠GAF = 35° (AC being the bisector)
⇒ ∠AEF = ∠AGF = 40° (Proved above)
∴ Δ AEF ~ Δ AGF (By A.A. axiom)
Hence, proved that Δ AEF ~ Δ AGF.
(b) In Δ ACD,
⇒ ∠CAD = 35°
⇒ ∠ADC = 40°
By angle sum property of triangle,
⇒ ∠CAD + ∠ADC + ∠DCA = 180°
⇒ 35° + 40° + ∠DCA = 180°
⇒ ∠DCA + 75° = 180°
⇒ ∠DCA = 180° - 75° = 105°.
In Δ ACD and Δ ACB,
⇒ ∠ACB = ∠ACD = 105° (Proved above)
⇒ ∠BAC = ∠DAC = 35° (Proved above)
∴ Δ ACD ~ Δ ACB (By A.A. axiom)
(i) Given,
⇒ AE : EB = 2 : 3
Let AE = 2x and EB = 3x.
∴ AB = AE + EB = 2x + 3x = 5x.
We know that,
Ratio of corresponding sides are proportional.
Hence, AG : AD = 2 : 5.
(ii) In △ ABC and △ ADC,
⇒ ∠BAC = ∠DAC (Both equal to 35°)
⇒ ∠ACB = ∠ACD (Both equal to 105°)
⇒ ∠ABC = ∠ADC (Both equal to 40°)
∴ △ ABC and △ ADC are congruent.
We know that,
Area of congruent triangles are equal.
Let area of △ ABC and area of △ ADC = x.
∴ Area of △ ABC : Area of △ ADC = x : x = 1 : 1.
Hence, area of △ ABC : area of △ ADC = 1 : 1.
(iii) From figure,
Area of quadrilateral ABCD = Area of △ ABC + Area of △ ADC = x + x = 2x.
∴ Area of quadrilateral ABCD : Area of △ ACB = 2x : x = 2 : 1.
Hence, area of quadrilateral ABCD : area of △ ACB = 2 : 1.
In the given figure, angle ABC = 70° and angle ACB = 50°. Given, O is the centre of the circle and PT is the tangent to the circle. Then calculate the following angles
(a) ∠CBT
(b) ∠BAT
(c) ∠PBT
(d) ∠APT
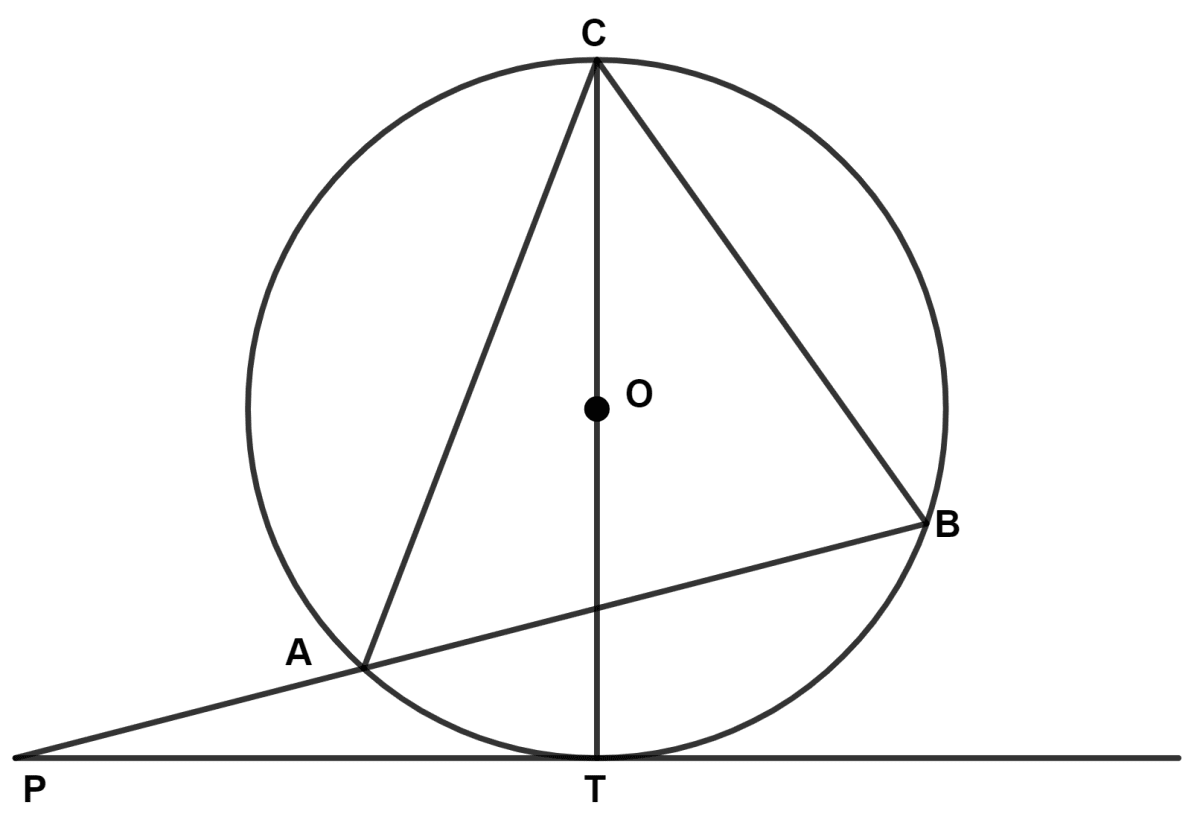
Answer
Join AT and BT.
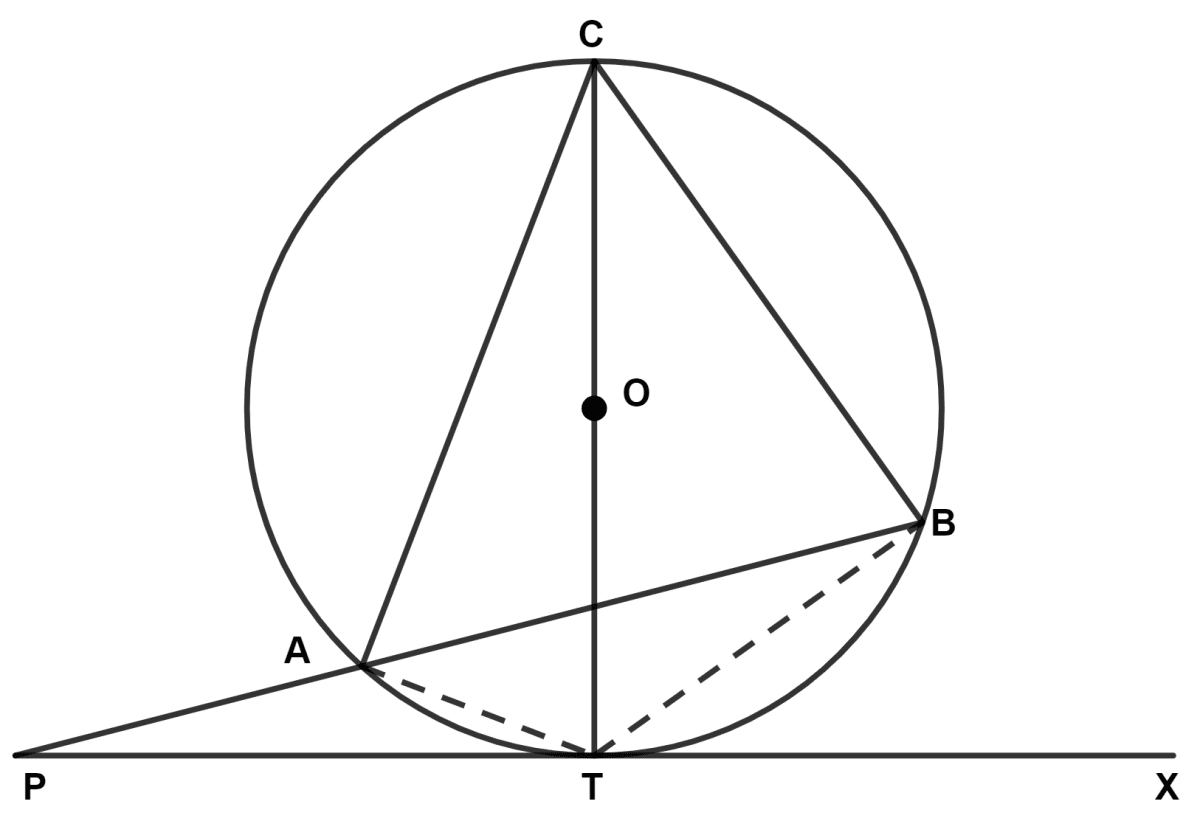
(a) We know that,
Angle in a semicircle is a right angle.
∴ ∠CBT = 90°.
Hence, ∠CBT = 90°.
(b) In cyclic quadrilateral ATBC,
⇒ ∠CBT + ∠CAT = 180° (∵ Sum of opposite angles of a cyclic quadrilateral = 180°)
⇒ 90° + ∠CAT = 180°
⇒ ∠CAT = 180° - 90° = 90°.
In △ABC,
⇒ ∠CBA + ∠CAB + ∠ACB = 180° [By angle sum property of triangle]
⇒ 70° + ∠CAB + 50° = 180°
⇒ ∠CAB + 120° = 180°
⇒ ∠CAB = 180° - 120°
⇒ ∠CAB = 60°.
From figure,
∠BAT = ∠CAT - ∠CAB = 90° - 60° = 30°.
Hence, ∠BAT = 30°.
(c) From figure,
∠BTX = ∠BAT = 30° [Angle in same segment are equal]
∠PBT = ∠CBT - ∠CBA = 90° - 70° = 20°.
Hence, ∠PBT = 20°.
(d) Since, ∠PTB and ∠BTX are linear pairs.
⇒ ∠PTB = 180° - ∠BTX = 180° - 30° = 150°.
In △PBT,
⇒ ∠PBT + ∠PTB + ∠APT = 180° [By angle sum property of triangle]
⇒ 20° + 150° + ∠APT = 180°
⇒ ∠APT + 170° = 180°
⇒ ∠APT = 180° - 170°
⇒ ∠APT = 10°.
Hence, ∠APT = 10°.
(a) Construct a triangle ABC such that BC = 8 cm, AC = 10 cm and ∠ABC = 90°.
(b) Construct an incircle to this triangle. Mark the centre as I.
(c) Measure and write the length of the in-radius.
(d) Measure and write the length of the tangents from vertex C to the incircle.
(e) Mark points P, Q and R where the incircle touches the sides AB, BC, and AC of the triangle respectively. Write the relationship between ∠RIQ and ∠QCR.
(Use a ruler and a compass for this question.)
Answer
Steps of construction :
Draw a line segment BC = 8 cm.
Draw BX perpendicular to BC.
With C as center and radius = 10 cm, draw an arc cutting BX at A.
Join AB and AC.
Draw AW, BY and CZ the angle bisectors of A, B and C respectively.
Mark the point of intersection as I.
Draw IR perpendicular to side AC.
With I as center and radius IR draw a circle, which is the required incircle.
Mark points P, Q and R where the incircle touches the sides AB, BC, and AC of the triangle respectively.
Measure CQ and CR.
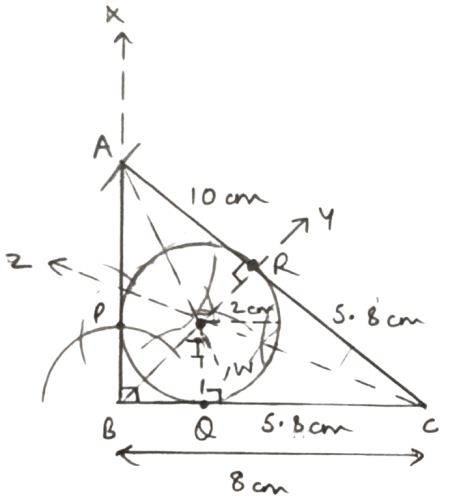
From figure,
⇒ ∠IRC = ∠IQC = 90° (The radius from the center of the circle to the point of tangency is perpendicular to the tangent line.)
⇒ ∠RCI = ∠QCI = (As CZ is angle bisector)
In △ IRC,
⇒ ∠RIC = 180° - ∠RCI - ∠IRC [∵ Sum of ∠'s in a Δ = 180°]
⇒ ∠RIC = 180° - - 90°
⇒ ∠RIC = 90° - ............(1)
In △ IQC,
⇒ ∠QIC = 180° - ∠IQC - ∠ICQ [∵ Sum of ∠'s in a Δ = 180°]
⇒ ∠QIC = 180° - 90° -
⇒ ∠QIC = 90° - ............(2)
Adding equations (1) and (2), we get :
⇒ ∠RIC + ∠QIC = 90° - + 90° -
⇒ ∠RIQ = 180° - ∠C
⇒ ∠RIQ = 180° - ∠RCQ
⇒ ∠RIQ + ∠RCQ = 180°.
Hence, ∠RIQ + ∠QCR = 180°.
The daily wages of workers in a construction unit were recorded as follows :
| Class marks (Wages) | No. of workers |
|---|---|
| 425 | 6 |
| 475 | 12 |
| 525 | 15 |
| 575 | 17 |
| 625 | 7 |
| 675 | 13 |
Form a frequency distribution table with class intervals and find modal wage by plotting a histogram.
Answer
Difference between two consecutive class marks = 475 - 425 = 50.
Adjustment factor = = 25.
Lower class limit = Class mark - Adjustment factor
Upper class limit = Class mark + Adjustment factor
| Class | Frequency |
|---|---|
| 400-450 | 6 |
| 450-500 | 12 |
| 500-550 | 15 |
| 550-600 | 17 |
| 600-650 | 7 |
| 650-700 | 3 |
Steps of construction :
Take 2 cm along x-axis = ₹50 and 2 cm along y-axis = 5 workers.
Construct rectangles corresponding to the given data.
In highest rectangle, draw two st. lines AD and BC from corners of the rectangles on either side of the highest rectangle to the opposite corners of the highest rectangle.
Let K be the point of intersection of AD and BC. Through K, draw a vertical line to meet the x-axis at L. The abscissa of the point L represents 557.50.
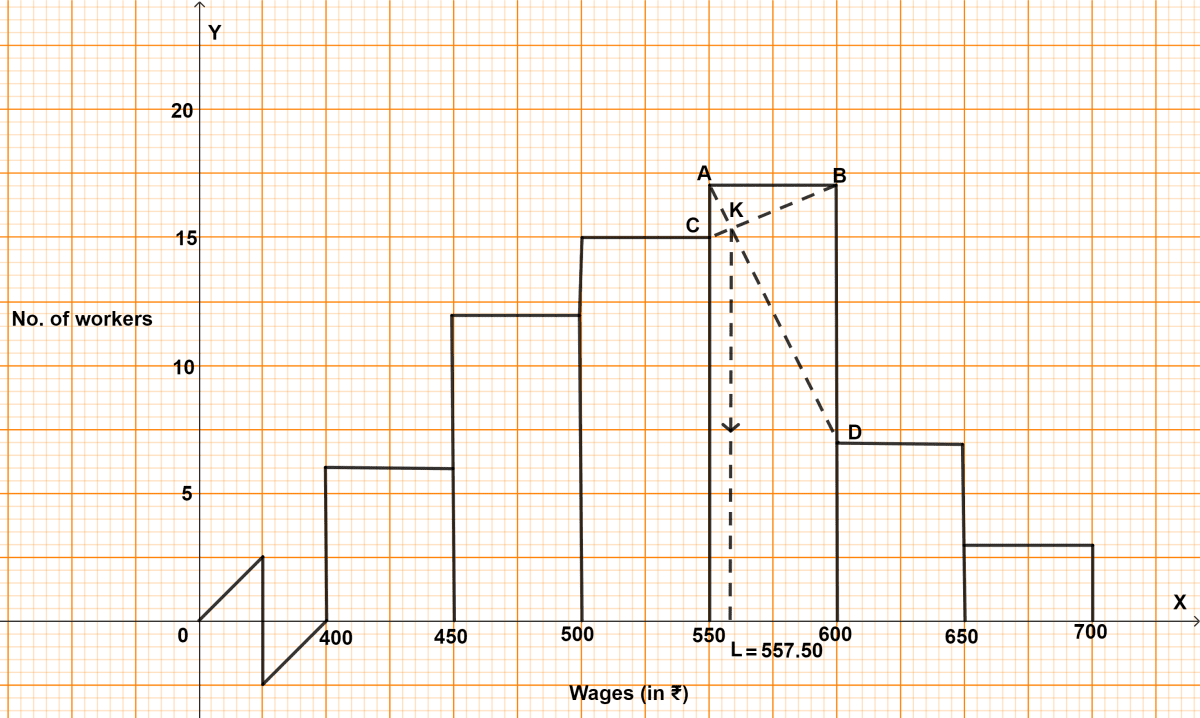
Hence, mode = ₹ 557.50
A bag contains 13 red cards, 13 black cards and 13 green cards. Each set of cards are numbered 1 to 13. From these cards, a card is drawn at random. What is the probability that the card drawn is a:
(a) green card?
(b) a card with an even number?
(c) a red or black card with a number which is a multiple of three?
Answer
Total no. of cards = 13 + 13 + 13 = 39 cards.
(a) P(that card drawn is a green card) = .
Hence, probability that card drawn is a green card = .
(b) Even number cards are : 2, 4, 6, 8, 10, 12.
So, there are 6 even cards of each set.
∴ 18 cards.
P(that card drawn is a card with even number)
= .
Hence, probability that card drawn is a card with even number = .
(c) Multiples of three : 3, 6, 9, 12.
So, there are 4 cards of each red and black colour.
∴ 8 cards.
P(that card drawn is a red or black card with multiple of three)
= .
Hence, probability that card drawn is a red or black card with multiple of three = .